One of the things I think scientists have to be guilty of is oversimplification – people just aren’t good at handling complex problems (why else is multivariable calculus so hard? For me?), so trying to simplify a problem down to just one variable is probably the easiest thing we can try to make way in science. Of course, the problem is that we sometimes overextrapolate, and we end up thinking a test tube is a human being (Figure 1).
One of the things biologists forget is that the things we study exist in real space. I know some of you think physicists are definitely guilty of this (Dude, seriously: a frictionless sphere is not a reasonable approximation for an elephant on fire. And why did you light the elephant on fire? Monsters.), but biologists are guilty of this, too, and probably more so. An electron in a vacuum isn’t really just an electron in a vacuum? Try a white blood cell on the hunt to murder some invading bacteria inside a thick, meaty, complicated, sweaty human – that ain’t no test tube. That white blood cell has to navigate a bunch of spaces in your body: your highly turbulent blood flow, the tight junctions between cells, whatever mess your liver is, etc. As such, a lone white blood cell encounters highly variable environments, especially pressure (Figure 2).

This brings me to one of my favorite papers that investigated how cells respond to resistance (“Biased migration of confined neutrophil-like cells in asymmetric hydraulic environments,” PNAS 2013). A neutrophil is one of your run-of-the-mill white blood cells – it hangs around your blood, but when there is some sort of injury or pathogen, the neutrophil is a first responder. Once it arrives on the scene, it can eat the enemy, shoot some antimicrobial torpedoes, or in a desperation/limit break move, throw its genome at the enemy to trap it in a mess of DNA (this is cleverly called a NET, or neutrophil extracellular trap). BANANAS!
But how does it get there? How does it get from your blood to the wad of bacterium that’s in your skin when you failed your olly and got some gnarly road rash? We used to put a lot of stock in chemotaxis – the idea that cells can figure out where to go based on a trail of chemicals. And that’s still true, but the authors of this paper also figured out that cells make decisions based on how tight the path they’re traveling in.
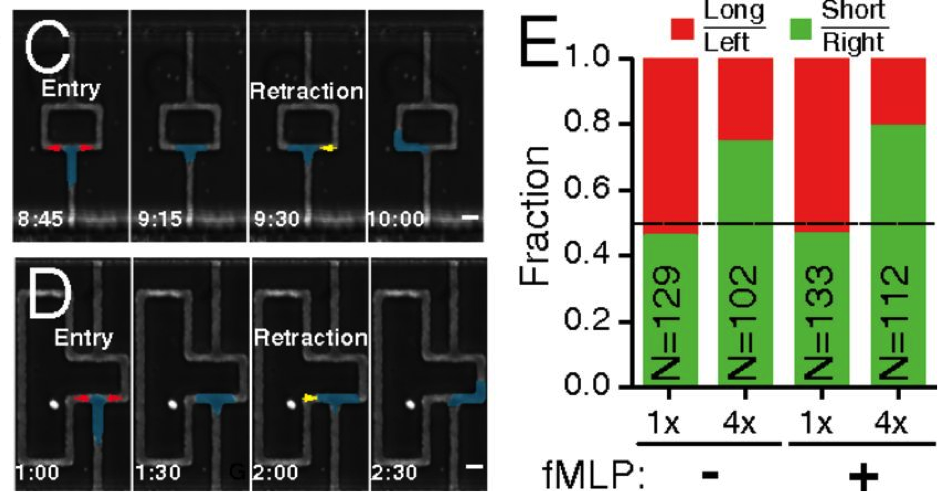
In Figure 3 (Panel C), you can see that the authors built a microfluidic system that’s a tight space for the neutrophil to travel in, where buffer is flowed from the top of the image to the bottom, and the cell starting at the bottom of the picture, resulting in a flow rate against the direction the cell can travel. In the top left panel, you can see that the cell (color coded in blue, yellow or red) has a choice – it can go left, or it can go right. In the 1st picture in Panel C, you can see the cell is extending into each branch – left and right – marked as red. So it’s the same cell, but red marks that the cell is stretching into that area. In the second picture, you can see the cell is now fully at that branch point. In the 3rd picture, now you see it’s made a decision. The yellow marks where the cell is now retracting its appendage, and in the last picture, you can see that the cell is now committed to the left path.
Each path has the same amount of hydraulic pressure, so you would imagine that it’s a flip of a coin on which way the cell chooses to go. Looking at Panel E, you can see in the first bar graph, it’s about a 50/50 split whether or not the cell goes left or right.
Now look at Panel D: the path is longer on the left side. Because the flow is continuous from the top to the bottom of the picture, the buffer on the longer path has to travel faster than the buffer on the right because the buffer is flowing at a constant rate. So not only is the path longer, but there’s also more liquid flowing downhill. If it were me, and I had to go from the bottom of the picture to the top of the picture, I would choose the shorter path with less resistance. And you can see that it does – again, in the first picture, you see once it gets to the branch point, it stretches out to feel out both directions. Then you see that it once again retracts the left part of the cell and commits in the rightward direction – the branch that has less resistance. Going back to Panel E, you can see that the cell makes the same decision: more than 50% of the time, it chooses the right side – the path of least resistance.
Interestingly, the researchers tried to lure the cell to the longer side by activating a chemotactic cue named fMLP – you can see the activation in the little dot on all the images in Panel D. Neutrophils run to fMLP like a cat to a precariously perched vase. But the cell doesn’t seem to care whether or not fMLP is there – it is choosing the path of least resistance.
Now if you were a good scientist, you might think – wait a minute. How does the cell know that it’s the path of least resistance, and not just the shorter path?
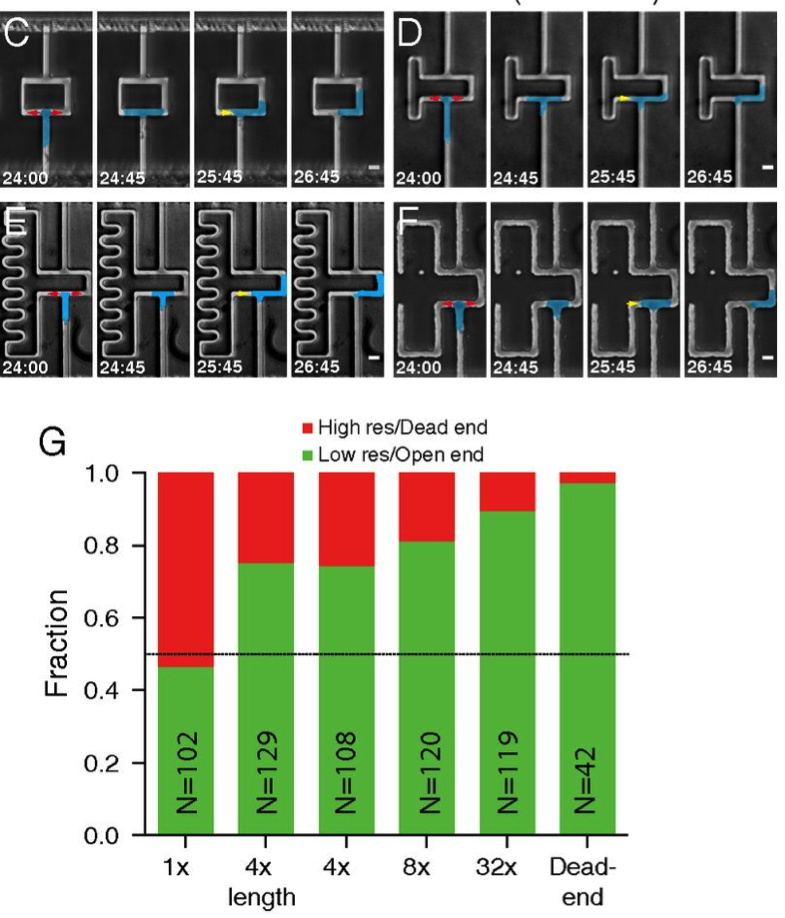
Behold Figure 4. Panel C addresses that exact question: what if you built them of the same length, but made the width shorter, thereby increasing the resistance in the same length. Boom. Still chooses the path of least resistance (Panel G). And the tougher you make it, the more the cell is likely to choose the easiest side. And if one side is a dead end? Forget it.
This opens up huge questions – immunologists like to consider cell migration primarily as a function of chemical cues, but what about physical cues, like pressure? When you injure yourself, you get a whole bunch of swelling because all your vessels become leaky so your defense systems have better access, but that would mean that there’s going to be higher turgor pressure all throughout. How do cells compensate for this? Do cells respond differently to different pressures? We know that stem cells can turn into bone, muscle, or epitheilal like cells depending on how hard the surface they’re living on are, so we know that at least some cells can respond differently to different pressures. We also know that things like oxygen levels can cause cells to respond differently. What about other gases? Osmolytes? Sound? Light? Electromagnetic signals?

Check out this turtle that Dr. David Gruber pictured (http://www.davidgruber.com/turtles/). IT IS FLUORESCENT. WHY!? Light is pretty garbage in water compared to light in the air, so why would the turtle fluoresce? Is it just an accident of biology? Or is there a need for a fluorescing turtle? Or frogs? Or fluorescing scorpions? Or bird bills?
Someone needs to find out why. Macroscale biophysics needs to be a thing.